Is the Gulf Stream Slowing Down? A Mathematical Perspective

By John Bailie

The ocean’s global currents are constantly circulating energy and nutrients worldwide. Photo by Andreas Lindgren on Unsplash.

The Atlantic Meridional Overturning Circulation (AMOC) is a large conveyer belt of water responsible for the Gulf Stream, generating a warmer Europe by northward heat transport. Warm surface currents transport water northward to the Labrador and Nordic seas. The water becomes denser and sinks in deep water formation regions. Mixing occurs in these regions between surface and deep waters. A deep cold current transports deepwater southward via the North Atlantic Deep Water (NADW) current. Southern upwelling closes the circulation; for more information, see Fig. 1(a) and [6].

Observed in the last century is an unprecedented slowdown of the AMOC [11]. The slowdown has been linked to North Atlantic freshening in the late 1900s in an event called the Great Salinity Anomaly (GSA) [3]. Moreover, increased freshwater influx resulted in the Labrador sea convection shutting down from 1969 to 1971, weakening the AMOC.

Freshwater in the North Atlantic could become comparable to the GSA if the current fresh-water trend from melting of the Greenland Ice Sheet continues [1]. As a result, a weaker AMOC and another shutdown of deepwater formation in the Labrador Sea is possible [11].

A slower AMOC could weaken the Gulf Stream and result in temperature drops in Europe, which would be more severe during winter. Precipitation in Europe could decrease, and possibly due to drier conditions, vegetation would also fall [5]. Moreover, the North Atlantic marine ecosystem could decline, and global plankton production could decrease significantly [12].

My Master’s project with Prof. Bernd Krauskopf at the Mathematics Department aims to understand the effect of freshwater entering the AMOC as represented by a much simplified conceptual climate model. The project is in collaboration with Prof. Henk A. Dijkstra at Utrecht University.

Modelling

Climate systems are large, with many variables that determine the overall state. Modelling approaches vary and consider different inner processes. General Circulation Models (GCMs) are large and discretise the full climate system on a fine-scale but are black boxes that are very hard to study. Zonally Averaged Models use far fewer variables than GCMs but still account for wind stress and the Earth’s rotation. While more tractable, it is still hard to understand the system’s underlying mechanisms [4].

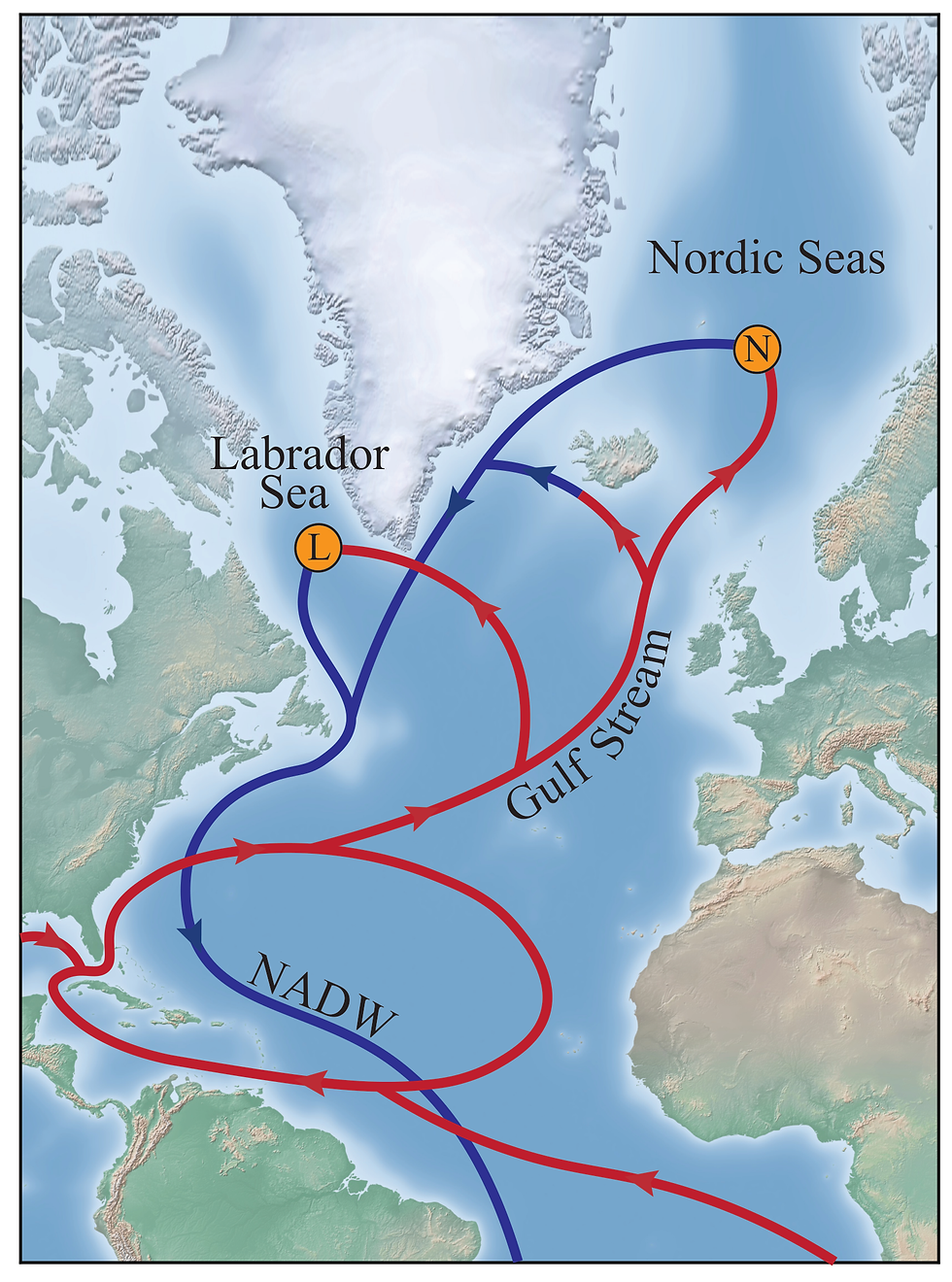
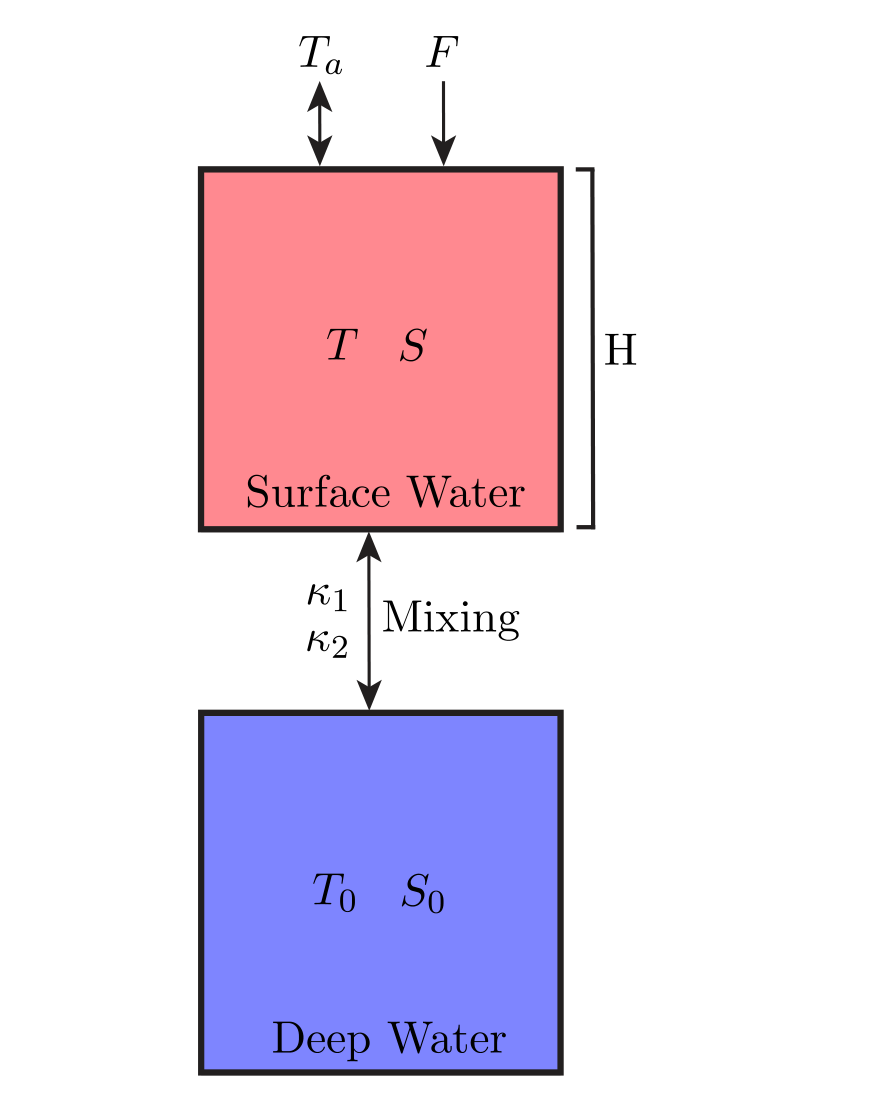
Figure 1: (a) The North Atlantic component of the AMOC with deep water formation sites at L and N in the Labrador and Nordic seas. The background is generated with the software from [8] and ocean currents are illustrated following from [10]. (b) Two-box model for temperature and salinity in the surface and deepwater layers at sites L and N.

Box models only consider a few variables in a relatively small number of boxes of concern. They are generally not used for prediction but are relatively simple and can be readily analysed. Box models are useful for understanding the underlying mechanisms of a physical process in isolation but are still a part of the larger climate system. Welander’s model [13] is a box model for temperature and salinity in only two boxes; a surface box and a deep water box that interact through mixing. A surrounding basin also interacts with the surface box; see Fig. 1(b). For certain ranges of freshwater intake, there are oscillations between temperature and salinity. Welander’s model was re-examined in [2] with the surrounding basin being replaced with a stochastic freshwater influx. More recent work was performed in [7] to formalise Welanders result by using a modern approach to piecewise smooth dynamical systems. However, a comprehensive classification of the dynamics with respect to freshwater influx is still missing, and this is the subject of my project.

As a starting point, we look at a limiting case of two decoupled differential equations, one for weak and one for strong mixing between two boxes. Because transitions between mixing states are assumed to be instantaneous, the overall model is piecewise smooth. As a result, we use numerical methods adapted from [9] to study its bifurcations and dynamics.

Results

So far, we have obtained a full description of all possible dynamics, organised by changes in the freshwater influx and the density difference between boxes. All possible states the AMOC can take in our model are described, and the mechanisms by which the AMOC transitions between them.

When the density of the surface box is much larger than the density of the bottom box, then strong mixing occurs between the layers, resulting in a stronger AMOC. Conversely, due to freshwater influx, the density of the surface box may become small enough for weak mixing to occur between the layers, resulting in a weaker AMOC.

The oscillations between temperature and salinity predicted in the literature [13, 2, 7] exist for ranges of freshwater and densities; see Fig. 2 for an illustration. During these oscillations, temperature and salinity both rapidly increase to a maximum value, then switch instantaneously to a slower relaxation; the process then repeats. The long-term behaviour of temperature and salinity from any initial condition approaches this type of oscillation. The green curve in Fig. 2(b) illustrates the oscillations in temperature and salinity.

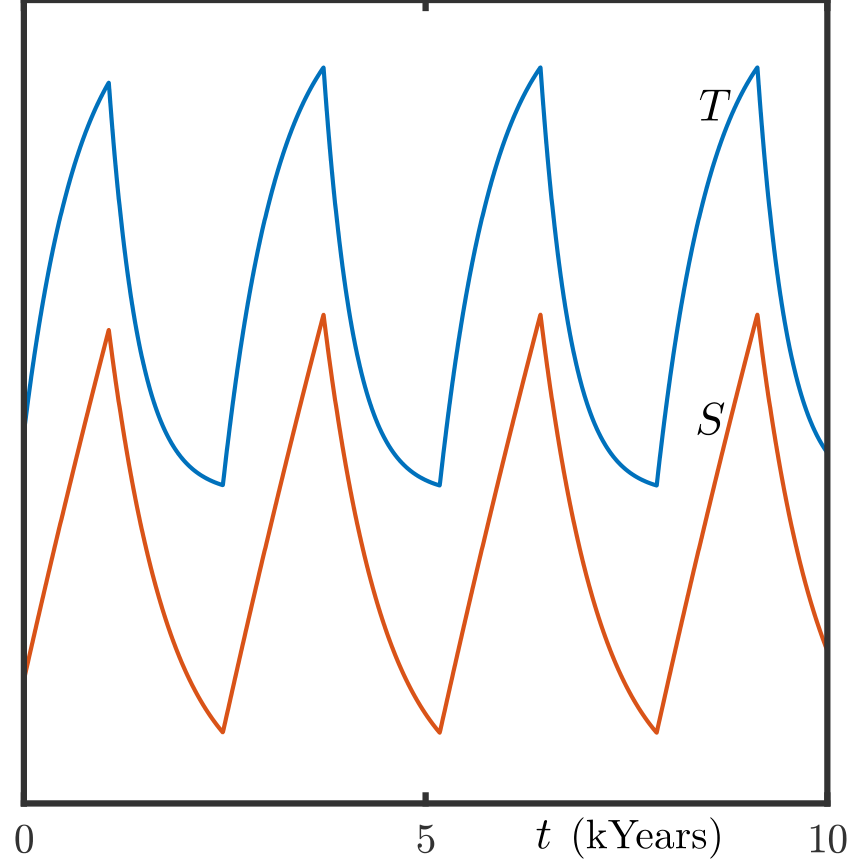
Figure 2: Oscilations of temperature T and salinity S, (a) as a time series and (b) in the (T,S) plane.

Conclusion and Outlook

The classification of the limiting case opens up the door to the study of the related smooth model. This smoothed case involves transitioning between strong and weak mixing in a slow-fast way rather than instantaneously. In particular, we expect the periodic behaviour between temperature and salinity to persist. The question is how these oscillations arise and disappear as parameters are varied in this more realistic context.

Future research will focus on expanding the current box model to make it more realistic. A first step will be adding a seasonal freshwater influx that changes periodically to account for seasonal fluctuations. The AMOC also displays delayed feedback loops of temperature and salinity. Incorporating these is an interesting challenge because it leads to a model in the class of delay differential equations. The analysis of which is more involved and results in a closer representation of the AMOC.

References

[1] Jonathan Bamber et al. “Recent large increases in freshwater fluxes from Greenland into the North Atlantic”. In: Geophysical Research Letters 39.19 (2012).

[2] Paola Cessi. “Convective adjustment and thermohaline excitability”. In: Journal of Physical Oceanography 26.4 (1996), pp. 481–491.

[3] Robert R Dickson et al. “The “great salinity anomaly” in the northern North Atlantic 1968–1982”. In: Progress in Oceanography 20.2 (1988), pp. 103–151.

[4] Henk A Dijkstra. Nonlinear physical oceanography: a dynamical systems approach to the large scale ocean circulation and El Nino. Vol. 28. Springer Science & Business Media, 2005.

[5] LC Jackson et al. “Global and European climate impacts of a slowdown of the AMOC in a high resolution GCM”. In: Climate dynamics 45.11 (2015), pp. 3299–3316.

[6] Till Kuhlbrodt et al. “On the driving processes of the Atlantic meridional overturning circulation”. In: Reviews of Geophysics 45.2 (2007).

[7] Julie Leifeld. Nonsmooth Homoclinic Bifurcation in a Conceptual Climate Model. 2016. arXiv: 1601.07936 [math.DS].

[8] Met Office. Cartopy: a cartographic python library with a matplotlib interface. Exeter, Devon, 2010 - 2015. URL: http://scitools.org.uk/cartopy.

[9] Petri T Piiroinen and Yuri A Kuznetsov. “An event-driven method to simulate Filippov systems with accurate computing of sliding motions”. In: ACM Transactions on Mathematical Software (TOMS) 34.3 (2008), pp. 1–24.

[10] Stefan Rahmstorf. “Risk of sea-change in the Atlantic”. In: Nature 388.6645 (1997), pp. 825–826.

[11] Stefan Rahmstorf et al. “Exceptional twentieth-century slowdown in Atlantic Ocean overturning circulation”. In: Nature climate change 5.5 (2015), pp. 475–480.

[12] Andreas Schmittner. “Decline of the marine ecosystem caused by a reduction in the Atlantic overturning circulation”. In: Nature 434.7033 (2005), pp. 628–633.

[13] Pierre Welander. “A simple heat-salt oscillator”. In: Dynamics of Atmospheres and Oceans 6.4 (July 1982), pp. 233–242. DOI: 10.1016/0377-0265(82)90030-6.